Rationalisation

In elementary algebra, root rationalisation is a process by which radicals in the denominator of an algebraic fraction are eliminated.

Rationalisation of the denominator:

Subject to x, y > 0,

1/(√x - √y) = (√x + √y)/{(√x - √y)(√x + √y)} = (√x + √y)/(x-y)

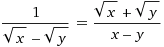
Rationalisation of the numerator:

Subject to x, y > 0,

√x - √y = (√x - √y)(√x + √y)/(√x + √y) = (x-y)/(√x + √y)

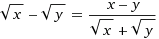
Double rationalisation: